Physics Notes

Topic 1 - Measurement and uncertainties

1.1 - Measurements in Physics

Fundamental units in SI system

Mass	Measured in kilograms (kg)
Length	Measured in meters (m)
Time	Measured in seconds (s)
Temperature	Measured in kelvin degrees (k)
Electric Current	Measured in amperes (A)
Luminosity	Measured in candela (cd)
Mole	Measured in moles (mol)

Scientific notation and metric multipliers

Power of 10	Prefix Name	Symbol
10^-12	pico	p
10^-9	nano	n
10^-6	micro	µ
10^-3	milli	m
10^-2	centi	c
10³	kilo	k
10⁶	mega	M
10⁹	giga	G
10¹²	tera	T

Significant figures

Description	Example	Significant Figures
All non-zero digits are significant	438 g	3
	26.42 m	4
	0.75 cm	2
All zeros between non-zero digits are significant	12060 m	4
All zeros between non-zero digits are significant	900.43 cm	5
Filler zeros to the left of an understood decimal place are not significant	220 L	2
	60 g	1
	30. cm	2
Filler zeros to the right of a decimal place are not significant	0.006 L	1
	0.08 g	1
All non-filler zeros to the right of a decimal place are significant	8.0 L	2
	60.40 g	4

1.2 - Uncertainties and errors

Random and systematic errors

Random error is error due to the recorder, rather than the instrument used for the measurement.

Different people may measure the same line slightly differently. You may in fact measure the same line differently on two different occasions.
Perhaps the ruler wasn't perfectly lined up.
Perhaps your eye was viewing at an angle rather than head-on.

Systematic error is error due to the instrument being "out of adjustment"

A voltmeter might have a zero offset error.
A meter stick might be rounded on one end.

Absolute, fractional and percentage uncertainties

Absolute error is the raw uncertainty or precision of your measurement.

Question:

A student measures the length of a line with a wooden meter stick to be 11 mm ± 1 mm. What is the absolute error or uncertainty in her measurement?

Solution:

The ± number is the absolute error. Thus 1 mm is the absolute error
1 mm is also the raw uncertainty

Fractional Error is given by:

Fractional Error

Fractional Error = \frac{Absolute Error}{Measured Value}

Question:

A student measures the length of a line with a wooden meter stick to be 11 mm ± 1 mm. What is the fractional error or uncertainty in her measurement?

Solution:

Fractional Error = \frac{1}{11} = 0.09

Percentage Error is given by:

Percentage Error

Percentage Error = \frac{Absolute Error}{Measured Value} \times 100%

Question:

A student measures the length of a line with a wooden meter stick to be 11 mm ± 1 mm. What is the percentage error or uncertainty in her measurement?

Solution:

Percentage Error = \frac{1}{11} \times 100% = 9%

Propagating uncertainties through calculations

To find the uncertainty in a sum or difference, you just add the uncertainty of all the ingredients.

Uncertainty in sums and differences

y = a \pm b

then

Δy = Δa + Δb

Question:

A 9.51 ± 0.15 meter rope ladder is hung from a roof that is 12.56 ± 0.07 meters above the ground. How far is the bottom of the ladder from the ground?

Solution:

$y = a - b = 12.56 - 9.51 = 3.05 m$
$Δy = Δa - Δb = 0.15 + 0.07 = 0.22 m$
Thus the bottom is 3.05 ± 0.22 m from the ground.

To find the uncertainty in a product or quotient you just add the percentage or fractional uncertainties of all the ingredients.

Uncertainty in product and quotient

y = \frac{a \times b}{c}

then

\frac{Δy}{y} = \frac{Δa}{a} + \frac{Δb}{b} + \frac{Δc}{c}

Question:

A car travels 64.7 ± 0.5 meters in 8.65 ± 0.05 seconds. What is its speed?

Solution:

Use rate = divided by time
r = d / t = 64.7 / 8.65 = 7.48 m s^-1
Δr / r = Δd / d + Δt / t = 0.5 / 64.7 + 0.05 / 8.65 = 0.0135
Δr / 7.48 = 0.0135
Δr = 7.48 × 0.0135 = 0.10 m s^-1
Thus, the car is travelling at 7.48 ± 0.10 m s^-1

1.3 - Vectors and scalars

Vector and scalar quantities

A vector quantity is one which has a magnitude (size) and a spatial direction.
A scalar quantity has only magnitude (size)

Topic 2 - Mechanics

2.1 - Motion

Distance and displacement

Distance is simply how far something has traveled witihout regard to direction.
Displacement, on the other hand, is not only distance traveled, but also direction

Displacement

Δ x = x_{2} - x_{1}

s = x_{2} - x_{1}

Where x₂ is the final position and x₁ is the initial position

Question:

Use the displacement formula to find each displacement. Note that the x = 0 coordinate has been placed on the number lines.

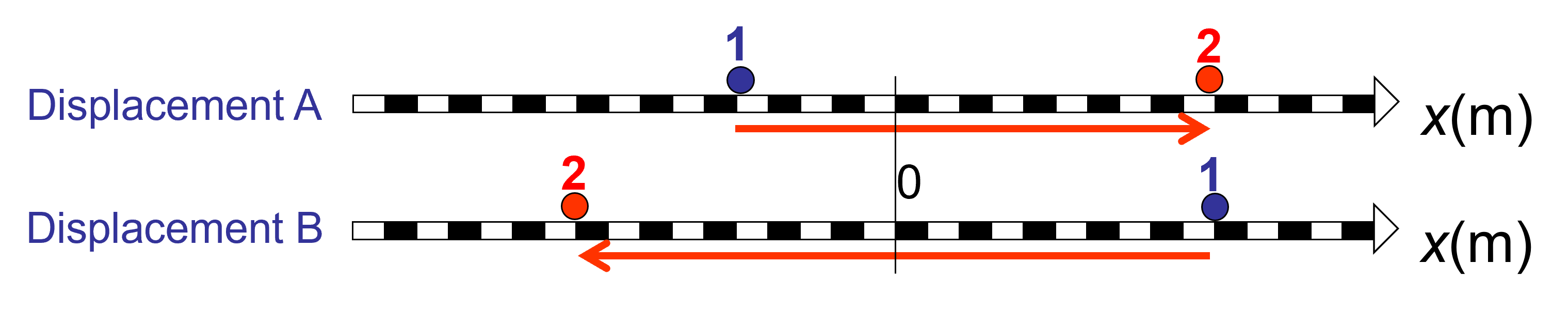
Solution:

For A: s = (+10) - (-5) = +15 m
For B: s = (-10) - (+10) = -20 m

Speed and velocity

Velocity v is a measure of how fast an object moves through a displacement
Thus, velocity is displacement divided by time, and is measured meters per second (m s^-1)

Velocity

v = Δ x / Δ t

v = s / t

Question:

A displacement of a ball is -20m. Find the velocity of the ball if it takes 4 seconds to complete its displacement.

Solution:

v = -20 m / 4 s = -5 m s^-1

Acceleration

Acceleration is the change in velocity over time.

Acceleration

a = Δ v / Δ t

a = (v - u) / t

Where v is the final velocity and u is the initial velocity

Question:

A driver sees his speed is 5.0 m s^-1. He then simultaneously accelerates and starts a stopwatch. At the end of 10 s he observes his speed to be 35 m s^-1. What is his acceleration?

Solution:

v = 35 m s^-1, u = 5.0 m s^-1, and t = 10 s
Use the formula: a = ( v − u ) / t
a = ( 35 − 5 ) / 10 = 3.0 m s^-2

Equations of motion for uniform acceleration

The equations for uniformly accelerated motion are also known as the kinematic equations

Kinematic equations

s = ut + (1/2)at²	Displacement
v = u + at	Velocity
v² = u² + 2as	Timeless
s = (u + v) t / 2	Average displacement

Solution:

Use the formula v = u + at
-90 = 50 + (-10)t
t = 14 s

Projectile motion

Kinematic Equations 2D

Δx = u_xt
v_x = u_x
Δy = u_yt + (1/2)a_yt²
v_y = u_y + a_yt

During projectile motion, the horizontal velocity is constant, when there is no air resistance. Thats why we can subtitude a = 0 when we are finding the horizontal component.